The infinite life of π
- Math Space BP. Malaysia
- Oct 1, 2022
- 4 min read
Abstract|
π has a variety of uses and it is vital in our daily life

What is π ?
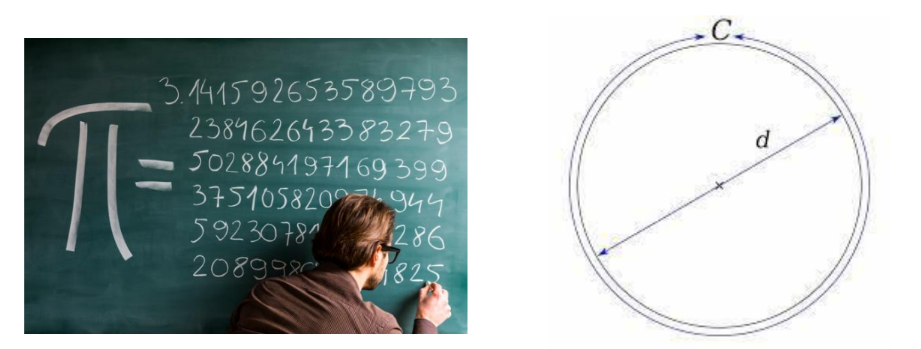
The number π, spelled out as “pi” is a mathematical constant that is the ratio of a
circle’s circumference to its diameter (π = c/d), approximately equal to 3.14159. The number
π mostly uses in formulas across mathematics and physics. As we all know, it is an irrational
number, meaning that it cannot be expressed exactly as integer or fraction, although
fractions such as 22/7 is commonly used to approximate it.
The History of π
The earliest historical record of pi can be traced back to about the 20th century BC, and
an ancient Babylonian stone plaque clearly recorded pi = 3.125. Ancient Egyptian artifacts
from the same period, Reind Mathematical Papyrus also showed that pi was equal to square
of the fraction 16/9, which is approximately equal to 3.1605.

It was not until the 3rd century BC that Archimedes, a famous mathematician and
physicist in ancient Greece, correctly calculated the Pi to three decimal places. After more
than 500 years, people promoted the π value from 3.141 to 3.14159 (Liu Hui, a Chinese
mathematician in the Wei and Jin Dynasties). More than 200 years later, Zu Chongzhi, a
mathematician in the Northern and Southern Dynasties, used the two numbers of surplus to
express the value of Pi between 3.1415926 and 3.1415927. The accuracy of π was calculated
to 7 decimal places, and the π value calculated by Zu Chongzhi was accurate for more than
800 years.

Until the early 15th century, the Arab mathematician Kassi obtained the 17 precise
decimal value of Pi, breaking the record maintained by Zu Chongzhi for nearly a thousand
years. German mathematician Ludolph van Ceulen calculated the π value to 20 decimal
places in 1596, and then devoted his life to it. In 1610, it was calculated to 35 decimal
places. This value was called Ludolph number in his name.
In ancient times, documents such as the Rhind Mathematical Papyrus found the area of
a circular shape in a curious, three-step manner:
* Find the diameter.
* Subtract the circle’s ninth part.
* Square the solution.
Ancient math teachers didn’t name their technique Pi, but essentially Pi was what they
used. Their value was higher than the one we use today, but it was close enough,
considering the tools they had to work with. Documents such as the Rhind Papyrus also
prove that children of ancient civilizations studied applied mathematics in much the same
way we do.
Not surprisingly, the ancient Egyptians were accountants and engineers who used
intricate mathematical formulas daily. The original Egyptian mathematical system topped
out at the one-million mark, still they could build impossible structures such as stone
pyramids, tombs and statues.
Uses of π
In basic mathematics, Pi is used for any calculations involving circles, from the volumn
of a can of soda to the orbits of satellites. And it is not just circles, it is also useful in studying
curves. Pi helps us understand periodic or oscillating systems like clocks, electromagnetic
waves and even music.
Some examples of daily uses of Pi:
1) Electrical engineers used pi to solve problems for electrical application
2) Statisticians use pi to track population dynamics
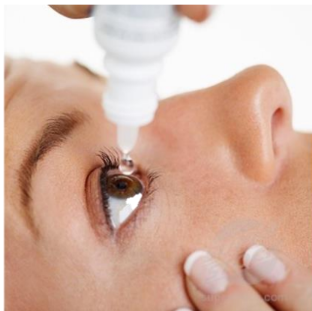
3) Medicine benefits from pi when studying the structure of the eye. This process is done to
produce people with eye medicine, such as eye drops
4) Biochemists see pi when trying to understand the structure/function of DNA
5) Physicists looking into the behavior of fluid ripples see pi and use it in their calculations
6) Clock designers use pi when designing pendulums for clocks
7) Aircraft designers use it to calculate areas of the skin of the aircraft
8) Signal processing and spectrum analysis (finding out what frequencies are in the wave
you are using) uses pi since the fundamental period of a sine wave is 2*pi.
9) Navigation, such as global positioning (GPS)
10)Calculating the number of death in a population
11) Solving Mathematics problems in Geometry like finding the area of circle etc.
Music & π
Music is certainly mathematical. Octaves. Fifths. Thirds. The western diatonic scale is a
mathematician’s paradise of interwoven frequencies. But here’s a question. Are pi and
music might be connected?
Pi is irrational. If we write it down — 3.14159... and so on — it goes on forever without
getting stuck in a repeating sequence of digits. Thus we might ask, if we assigned each digit
in pi to a key on the piano keyboard, say, "1" to middle C, "2" to D, and so on, what would
the music sound like? Listen for yourself.
https://youtu.be/wK7tq7L0N8E
by Michael Blake
The melody corresponds to the digits in pi. Actually, since a piano has twelve notes per
octave, not ten, the composer chose to write pi in base twelve. That way each note got its
own digit. The rhythm and harmony were the choice of the composer. Here’s a "jazz"
version where the composer let pi influence the rhythm and harmony as well as the melody.
It’s less structured, but still quite musical.

https://youtu.be/SNpab2PDquw
by Harlan Brothers
So what is it about pi that leads to such surprising music? Is there some mathematical
mystery behind what we hear?
In all likelihood, no. Pi isn’t random, but we’re pretty sure its digits occur randomly in a
well-defined sense. That means the melody they give rise to is similarly random. The allure
of the music doesn’t stem from some deep connection with numbers.
For more information:
D. Bailey and J. Borwein. Are the Digits of Pi Random? From the Huffington Post website:
http://www.huffingtonpost.com/david-h-bailey/are-the-digits-of-pi-
random_b_3085725.html.
A song of π from aSongScout
https://youtu.be/wM-x3pUcdeo
Why do we keep searching for more digits of pi?
-Computer testing. Supercomputers have a vast potential, and seeing how many digits of pi
it can find is a good way of finding out exactly how powerful its calculating potential is. The
first few digits are nice and easy, but as you go down it gets harder and harder.
-Our nature. Thousands of years ago in ancient Greece, pi was calculated to 3.14. What if
then, humans had said, “Ok, that’s good enough. Now let’s leave pi at that and move on to
other stuff.” Is that the nature of us inquiring humans? No, we want to have more and more
knowledge, become more precise and efficient. And that’s why we keep calculating the
digits of pi.
Reference:
https://en.wikipedia.org/wiki/Pi
AUTHOR

LYNLY TAN
Lynly is a form 3 student from SMK Dato' Bentara Luar, Batu Pahat, Johor.Being a math enthusiast,she aspires to pursue a profession that is connected with math in future to explore more about math


Comments